Chi chart square use please sample note only provided provide two problem suppose pasting luck question thank general good
Table of Contents
Table of Contents
Do you know what Chi Square Degrees Of Freedom Chart is? If you are working with statistics, particularly in social science research, you must have heard of this term at least once. But don’t worry: even if you are not a researcher, you can benefit from understanding what it is and how it works in statistical analysis. In this article, we will explain what Chi Square Degrees Of Freedom Chart is, why it is important, and how to use it.
Pain Points
Statistical analysis can be daunting, especially when dealing with complex concepts such as Chi Square Degrees Of Freedom Chart. Even experienced statisticians can have difficulties understanding it, let alone beginners. Moreover, there are often many different ways to approach a statistical problem, making it hard to choose the best method. Also, the results of statistical analysis can be misleading if the researcher doesn’t understand the data correctly, which can lead to incorrect conclusions.
Target of Chi Square Degrees Of Freedom Chart
Chi Square Degrees Of Freedom Chart is a statistical tool used to determine the independence between two categorical variables. In other words, it helps researchers to test whether two variables are related to each other or not. This method is particularly useful when dealing with non-numerical data and discrete categories. The Chi Square Degrees Of Freedom Chart shows the probability of obtaining the observed results under the assumption of independence between the variables. By comparing the obtained results with the expected ones, researchers can evaluate whether the two variables are independent or not.
Summary
In summary, Chi Square Degrees Of Freedom Chart is a statistical method used to test the independence between two categorical variables. It shows the probability of obtaining the observed results under the assumption of independence and compares them with the expected results. This tool is useful when dealing with non-numerical data and discrete categories.
Chi Square Degrees Of Freedom Chart: Explanation and Personal Experience
When I started my research project, I was surprised to find out that the most significant predictor of my dependent variable was the participant’s gender. However, I wasn’t sure whether gender was related to the independent variable or not. That’s when I stumbled upon Chi Square Degrees Of Freedom Chart. I had never used it before, but after reading some articles and watching some tutorials, I realized how helpful it could be.
Basically, Chi Square Degrees Of Freedom Chart allowed me to test whether the participant’s gender was independent of the independent variable. I calculated the chi-square value, and it turned out to be significant, indicating that gender and the independent variable were related. Thus, I had to control gender’s effect on the dependent variable while analyzing the data.
One thing to keep in mind is that Chi Square Degrees Of Freedom Chart can only test whether two variables are independent or not. It cannot prove causation, meaning that even if two variables are related, it doesn’t necessarily mean that one caused the other.
How to Use Chi Square Degrees Of Freedom Chart
Using Chi Square Degrees Of Freedom Chart involves several steps. First, formulate a research question with two categorical variables. Then, create a contingency table, where the rows represent one variable and the columns represent the other. The cells of the table represent the number of observations for each combination of the two variables.
Next, calculate the expected frequencies assuming independence between the variables. Then, calculate the chi-square value using the formula, and determine the degrees of freedom, which is the product of the number of rows minus 1 and the number of columns minus 1. You can compare the calculated chi-square value with the critical value of the chi-square distribution with the corresponding degrees of freedom and alpha level.
If the calculated chi-square value exceeds the critical value, you can reject the null hypothesis that the two variables are independent. You can also calculate the effect size, such as Cramer’s V, to determine the strength and direction of the association between the variables.
Why is Chi Square Degrees Of Freedom Chart Important?
Chi Square Degrees Of Freedom Chart is essential as it helps researchers to evaluate the independence between categorical variables. It is widely used in social science research, especially in fields such as psychology, sociology, and political science. By using Chi Square Degrees Of Freedom Chart, researchers can evaluate whether two variables are related or not, leading to more accurate and reliable research results.
Limitations
Although Chi Square Degrees Of Freedom Chart is a useful tool, it is not without limitations. It assumes that the observations are independent and random, the sample size is large enough, and the expected frequency is not less than five. The significance level can also be affected by the sample size, so caution is needed when interpreting the results.
Question and Answer
Q1: What types of data are suitable for Chi Square Degrees Of Freedom Chart?
A1: Chi Square Degrees Of Freedom Chart is suitable for non-numerical and categorical data, such as gender, race, religion, and political affiliation.
Q2: Can Chi Square Degrees Of Freedom Chart prove causation between two variables?
A2: No, Chi Square Degrees Of Freedom Chart can only test whether two variables are independent or not. It cannot prove causation.
Q3: What is the null hypothesis in Chi Square Degrees Of Freedom Chart?
A3: The null hypothesis is that the two variables are independent.
Q4: What is the degrees of freedom in Chi Square Degrees Of Freedom Chart?
A4: The degrees of freedom is the product of the number of rows minus 1 and the number of columns minus 1.
Conclusion of Chi Square Degrees Of Freedom Chart
Chi Square Degrees Of Freedom Chart is a statistical method used to test the independence between two categorical variables. This tool is essential in social science research, as it allows researchers to evaluate the relationship between two variables. By calculating the expected frequencies and comparing them with the observed ones, researchers can determine whether two variables are independent or not. However, Chi Square Degrees Of Freedom Chart has limitations, such as assumptions about the data and sample size. Caution is needed when interpreting the results.
Gallery
Chi-Square Distribution And Degrees Of Freedom - Programmathically
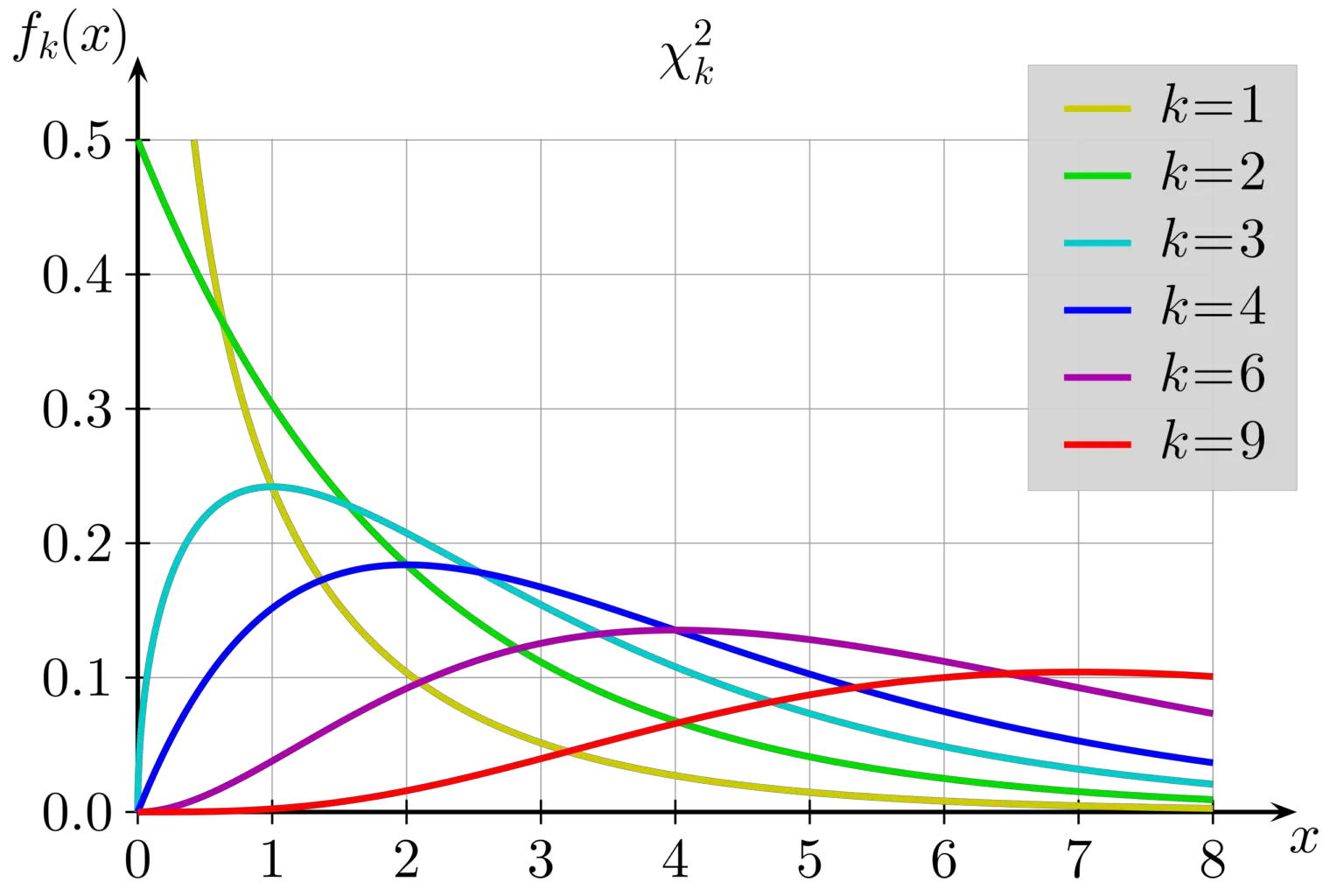
Photo Credit by: bing.com / distribution degrees distributions creativecommons
Chi-Square 1: Goodness Of Fit | Dr. Yury Zablotski

Photo Credit by: bing.com /
Solved Note: Please Only Use The Chi Square Chart Provided | Chegg.com
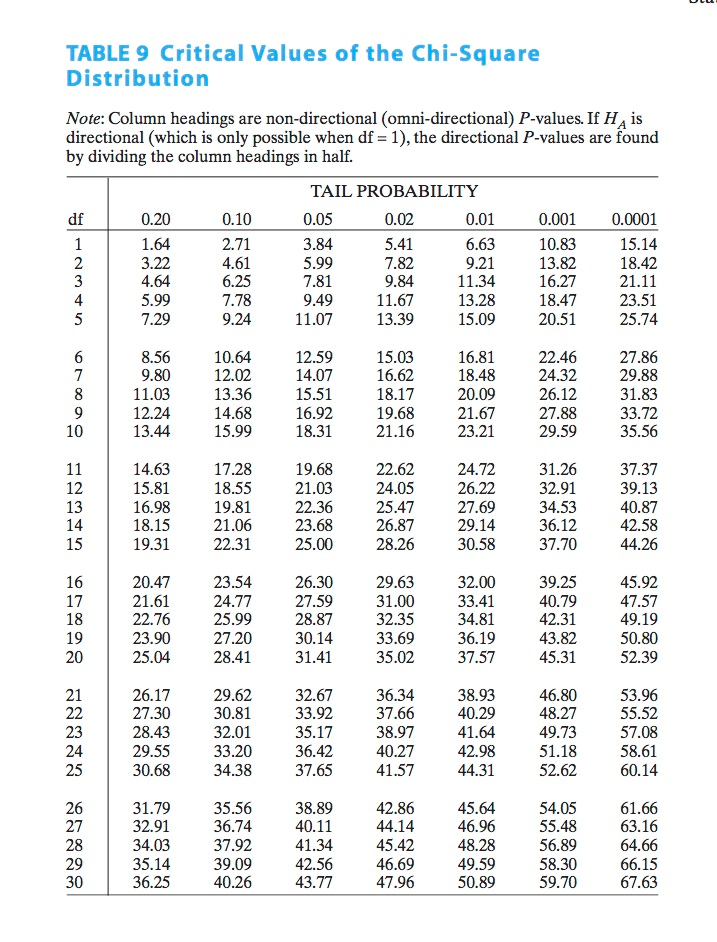
Photo Credit by: bing.com / chi chart square use please sample note only provided provide two problem suppose pasting luck question thank general good
Chi Square Distribution | Six Sigma Study Guide
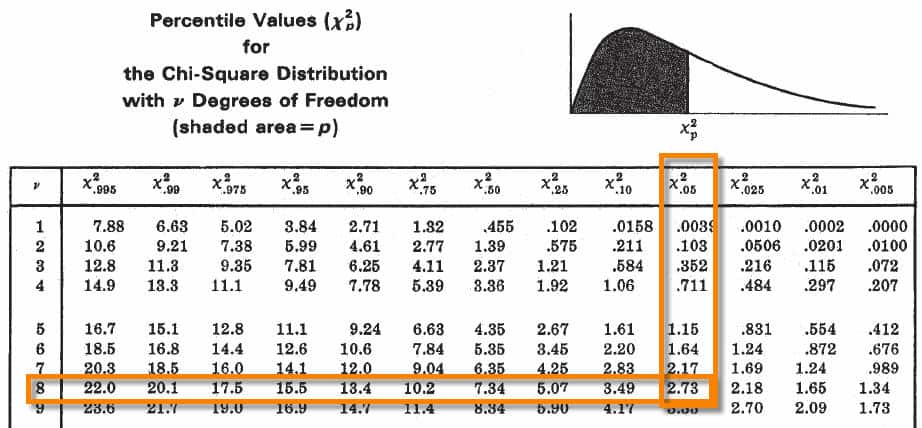
Photo Credit by: bing.com / chi square distribution chart test example hypothesis sigma variance use sample sixsigmastudyguide deviation ex1 tailed
NS Table D - Chi-square
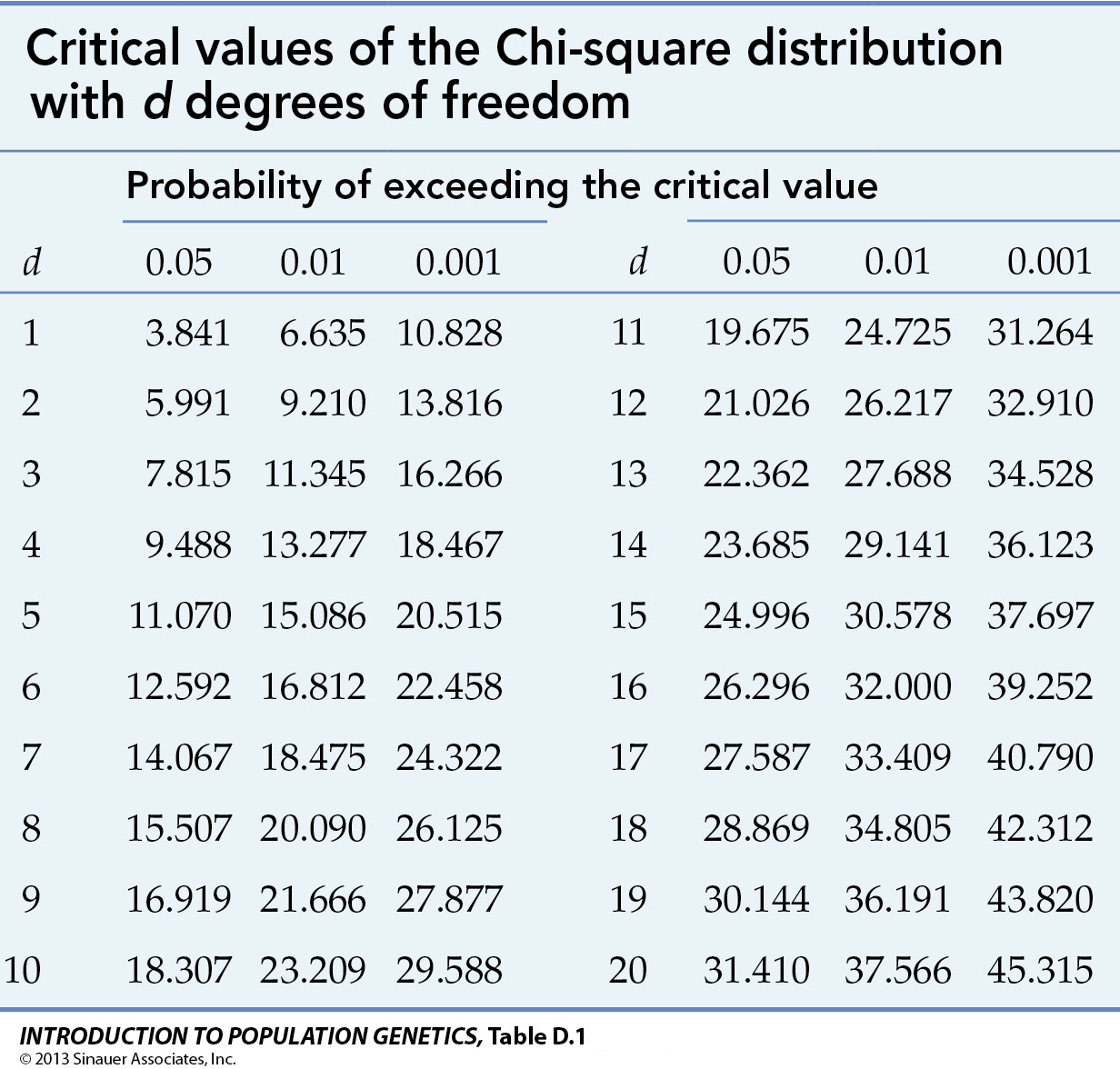
Photo Credit by: bing.com / chi square critical values table degrees distribution biology freedom